Aptitude Important Short Tricks to solve Simple Interest Questions

Important Short Tricks to solve Simple Interest Questions

To make the chapter easy for you all, we are providing you all some Important Short Tricks to solve Simple Interest Questions which will surely make the chapter easy for you all.

Important Short Tricks to solve Simple Interest Questions

Interest is the money paid by the borrow to the lender for the use of money lent.
The sum lent is called the Principal. Interest is usually calculated at the rate of so many rupees for every Rs.100 of the money lent for a year. This is called the rate per cent per annum.
‘Per annum’ means for a year. The words ‘per annum’ are sometimes omitted. Thus, 6 p.c. means that Rs.6 is the interest on Rs.100 in one year.
The sum of the principal and interest is called the amount. The interest is usually paid yearly, half-yearly or quarterly as agreed upon.
Interest is of two kind, Simple and Compound. When interest is calculated on the original principal for any length of time it is called simple interest. Compound interest is defined in the next chapter.

To find Simple Interest, multiply the principal by the number of years and by the rate per cent and divide the result by 100.

This may be remembered in the symbolic form

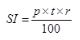
Where I = Interest, p = principle, t = number of years, r = % rate

Ex. 1. Find the simple interest on Rs.400 for 5 years at 6 percent.

Solution:

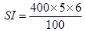
Interest for a number of days

When the time is given in days or in years and days, 365 days are reckoned to a year. But when the time is given in months and days, 12 months are reckoned to a year and 30 days to the month. The day on which the money is paid back should be include be but not the day on which it is borrowed, ie, in counting, the first day is omitted.

Ex. 2. Find the simple interest on Rs.306. 25 from March 3^rd to July 27^that

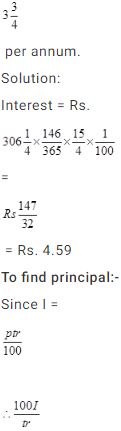
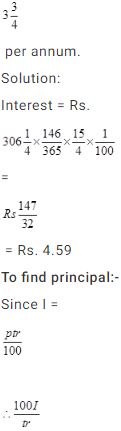
Ex.3. What sum of money will produce Rs.143 interest in

Ex. 4. A sum of Rs.468.75 was lent out at simple interest and at the end of 1 year 8 months the total amount was Rs500. Find the rate of interest per cent annum.

Solution:

Here, P =Rs468.75, t =

Ex. 5. In what time will Rs.8500 amount to Rs.15767.50 at

Miscellaneous Examples on Simple Interest:-

Ex.6: The simple interest on a sum of money is 1/9^th of the principal, and the number of years is equal to the rate per cent per annum. Find the rate per cent.

Solution:

Let principal = P, time = t year, rate = t

Then,

Ex. 7: The rate of interest for the first 2 yrs is 3% per annum, for the next 3 years is 8% per annum and for the period beyond 5 years 10% per annum.To fetch an interest of 1520 in six years, money did he deposit?

Solution

Let his deposit be = Rs 100

Interest for first 2 yrs = Rs 6

Interest for next 3 yrs = Rs 24

Interest for the last year = Rs 10

Total interest = Rs 40

When interest is Rs 40, deposited amount is Rs 100

when interest is Rs 1520, deposited amount =

 Ex.8: A sum of money doubles itself in 10 years at simple interest. What is the rate of interest?

Solution:

Let the sum be Rs 100

After 10 years it becomes Rs 200

Interest = 200 - 100 = 100

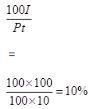
Direct formula:

Time × Rate = 100 (Multiple number of principal – 1)

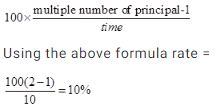
Ex.9: A sum was put at a certain rate for 2 yrs. Had it been put at 3% higher rate, it would have fetched Rs 300 more. Find the sum.

Solution:

Let the sum be Rs x and the original rate be y% per annum. Then, new rate = (y+3) % per annum

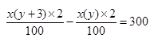
xy +3x – xy =15,000 or, x =5000

Thus, the sum =Rs 5000

Gradestack Method : Direct Formula

Sum =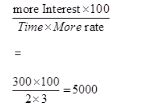 

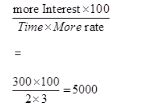
Ex.10. The simple interest on a certain sum of money at 4% per annum for 4 yrs is Rs 80 more than the interest on the same sum for 3 yrs at 5% per annum. Find the sum.

Solution:

Let the sum be Rs.x , then, at 4% rate for 4 yrs the simple interest =

We hope you all like the post. Don’t forget to share your feedback with us in the comment box.

To view the Important Short Tricks to solve Compound Interest Questions, click on the link given below: