Aptitude Important Mensuration (2D) Formulas

Important Mensuration (2D) Formulas

Important Formulas on Quadrilateral and Circle

Rectangle

A four-sided shape that is made up of two pairs of parallel lines and that has four right angles; especially: a shape in which one pair of lines is longer than the other pair.

The diagonals of a rectangle bisect each other and are equal.

Area of rectangle = length x breadth = l x b

OR Area of rectangle = 

if one sides (l) and diagonal (d) are given.

OR Area of rectangle = 

if perimeter (P) and diagonal (d) are given.

Perimeter (P) of rectangle = 2 (length + breadth) = 2 (l + b).

OR Perimeter of rectangle =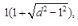 

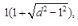
if one sides (l) and diagonal (d) are given.

Square

A four-sided shape that is made up of four straight sides that are the same length and that has four right angles.

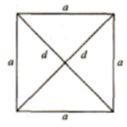
The diagonals of a square are equal and bisect each other at 90⁰.

(a) Area (a) of a square

Circle

A circle is the path traveled by a point which moves in such a way that its distance from a fixed point remains constant.

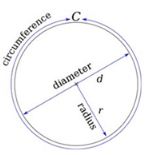
The fixed point is known as center and the fixed distance is called the radius.

(a) Circumference or perimeter of circle =

Sector :

A sector is  a figure enclosed by two radii and an arc lying between them.

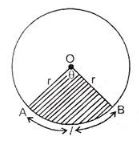
here AOB is a sector 

length of arc AB= 2πrΘ/360°

Area of Sector ACBO=1/2[arc AB×radius]=πr×r×Θ/360°

Ring or Circular Path:

R=outer radius

r=inner radius

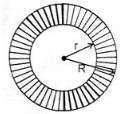
 area=π(R²-r²)

Perimeter=2π(R+r)

Rhombus

Rhombus is a quadrilateral whose all sides are equal.

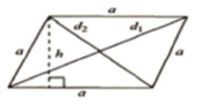
The diagonals of a rhombus bisect each other at 90⁰

Area (a) of a rhombus

= a * h, i.e. base * height

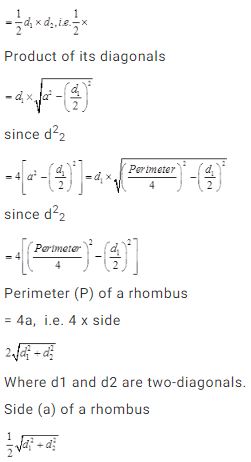
Parallelogram

A quadrilateral in which opposite sides are equal and parallel is called a parallelogram. The diagonals of a parallelogram bisect each other.

Area (a) of a parallelogram = base × altitude corresponding to the base = b × h

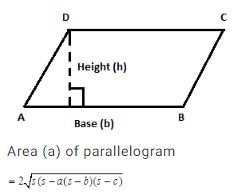
where a and b are adjacent sides, d is the length of the diagonal connecting the ends of the two sides and

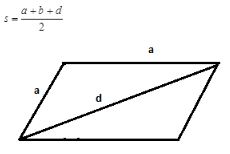
In a parallelogram, the sum of the squares of the diagonals = 2

(the sum of the squares of the two adjacent sides).

i.e.,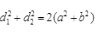 

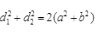
Perimeter (P) of a parallelogram

= 2  (a+b),

Where a and b are adjacent sides of the parallelogram.

Trapezium (Trapezoid)

A trapezoid is a 2-dimensional geometric figure with four sides, at least one set of which are parallel. The parallel sides are called the bases, while the other sides are called the legs. The term ‘trapezium,’ from which we got our word trapezoid has been in use in the English language since the 1500s and is from the Latin meaning ‘little table.’

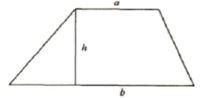
Area (a) of a trapezium

1/2 x (sum of parallel sides) x perpendicular

Distance between the parallel sides

i.e.,

​​​​​​​

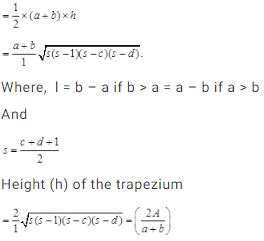
Pathways Running across the middle of a rectangle:

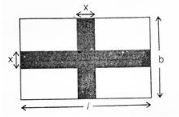
X is  the width of the path

Area of path= (l+b-x)x

perimeter=  2(l+b-2x)

Outer Pathways:

Area=(l+b+2x)2x

Perimeter=4(l+b+2x)

Inner Pathways:

Area=(l+b-2x)2x

Perimeter=4(l+b-2x)

Some useful Short trick:

• If there is a change of X% in defining dimensions of the 2-d figure then its perimeter will also changes by X%
• If all the sides of a quadrilateral is changed by  X% then its diagonal will also changes by X%.
• The area of the largest triangle that can be inscribed in a semi circle of radius r is r^2.
• The number of revolution made by a circular wheel of radius r in travelling distance d is given by

                         number of revolution =d/2πr

• If the length and breadth of rectangle are increased by x% and y% then the area of the rectangle will increased by.

                                (x+y+xy/100)%

• If the length and breadth of a rectangle is decreased by by x% and y% respectively then the area of the rectangle will  decrease by:

                                    (x+y-xy/100)%

• If the length of a rectangle is increased by x%, then its breadth will have to be decreased by (100x/100+x)% in order to maintain the same area of the rectangle.
• If each of the defining dimensions or sides of any 2-D figure is changed by x% its area changes by

          x(2+x/100)%

where x=positive if increase and negative if decreases.