Time & Work Concept, Tips & Tricks
TIPS FOR SOLVING QUESTIONS RELATED TO TIME AND WORK:
1. The total work is taken as 1.
2. If A can do a piece of work in 'n' days, ,then A's 1 day's work is equal to = 1/n
3. If A's 1 day's work = 1/n, then A can complete the work in 'n' days.
4. If A is thrice as good a workman as B, then
Ratio of work done by A and B = 3 : 1.
Ratio of time taken by A and B to finish the work = 1 : 3.
5.If ‘M1’ persons can do ‘W1’ work in ‘D1’ days and ‘M2’ persons can do W2 work in D2, days then we have a very general formula in the relationship of M1 D1 W2 = M2 D2 W1. The above relationship can be taken as a very basic and all-in-one formula. We also derive
A) More men less days and conversely more days less men.
B) More men more work and conversely more work more men.
C) More days more work and conversely more work more days.
6.If we include the working hours (say T1 and T2) for the two groups then the relationship is
M1 D1 T1 W2 = M2 D2 T2 Q1
Again, if the efficiency (Say E1 and E2) of the persons in two groups is different then the relationship is
M1 D1 T1 E1 W2 = M2 D2 T2 E2 W1
Now, we should go ahead starting with simpler to difficult and more difficult questions.
Questions on Above Formulaes
1.A alone can do a piece of work in 6 days and B alone in 8 days. A and B undertook to do it for Rs. 3200. With the help of C, they completed the work in 3 days. How much is to be paid to C?
A. Rs. 375
B. Rs. 400
C. Rs. 600
D.Rs. 800
ANSWERS AND SOLUTION :
1(B)Explanation:
C's 1 day's work = 1/3 - (1/6 + 1/8) = 1/3 - 7/24 = 1/24
A's wages : B's wages : C's wages = 1/6 : 1/8 : 1/24 = 4 : 3 : 1.
C's share (for 3 days) = Rs. (3 x 1/24 x 3200) = Rs. 400.
2. Machine P can print one lakh books in 8 hours. Machine Q can print the same number of books in 10 hours while machine R can print the same in 12 hours. All the machines started printing at 9 A.M. Machine P is stopped at 11 A.M. and the remaining two machines complete work. Approximately at what time will the printing of one lakh books be completed?
A. 3 pm
B. 2 pm
C. 1:00 pm
D. 11 am
ANSWERS AND SOLUTION :
2(C)Explanation :
Work done by P in 1 hour = 1/8
Work done by Q in 1 hour = 1/10
Work done by R in 1 hour = 1/12
Work done by P,Q and R in 1 hour = 1/8 + 1/10 + 1/12 = 37/120
Work done by Q and R in 1 hour = 1/10 + 1/12 = 22/120 = 11/60
From 9 am to 11 am, all the machines were operating.
Ie, they all operated for 2 hours and work completed = 2 × (37/120) = 37/60
Pending work = 1- 37/60 = 23/60
Hours taken by Q an R to complete the pending work = (23/60) / (11/60) = 23/11
which is approximately equal to 2
Hence the work will be completed approximately 2 hours after 11 am ; ie around 1 pm.
3. A is thrice as good as workman as B and therefore is able to finish a job in 60 days less than B. Working together, they can do it in:
A. 20 days
B. 22 1/2days
C. 25 days
D. 30 days
ANSWERS AND SOLUTION :
3(B)Explanation:
Ratio of times taken by A and B = 1 : 3.
The time difference is (3 - 1) 2 days while B take 3 days and A takes 1 day.
If difference of time is 2 days, B takes 3 days.
If difference of time is 60 days, B takes (3/2 x 60) = 90 days.
So, A takes 30 days to do the work.
A's 1 day's work = 1/30
B's 1 day's work = 1/90
(A + B)'s 1 day's work = (1/30 1/90) = 4/90 = 2/45
So A and B together can do the work in 45/2 = 22 1/2 days.
4. P is 30% more efficient than Q. P can complete a work in 23 days. If P and Q work together, how much time will it take to complete the same work?
A. 9
B. 11
C. 13
D. 15
ANSWERS AND SOLUTION :
4(C)Explanation :
Work done by P in 1 day = 1/23
Let work done by Q in 1 day = q
q × (130/100) = 1/23
=> q = 100/(23×130) = 10/(23×13)
Work done by P and Q in 1 day = 1/23 + 10/(23×13) = 23/(23×13)= 1/13
=> P and Q together can do the work in 13 days
5. If 6 men and 8 boys can do a piece of work in 10 days while 26 men and 48 boys can do the same in 2 days, the time taken by 15 men and 20 boys in doing the same type of work will be:
A. 4 days
B. 5 days
C. 6 days
D. 7 days
ANSWERS AND SOLUTION :
5(A)Explanation:
Let 1 man's 1 day's work = x and 1 boy's 1 day's work = y.
Then, 6x + 8y = 1/10 and 26x + 48y = 1/2,.
Solving these two equations, we get : x = 1/100 and y = 1/200 .
(15 men + 20 boy)'s 1 day's work = (15/100 + 20/200) = 1/4
So, 15 men and 20 boys can do the work in 4 days.
6.40 men can cut 60 trees in 8 hours. If 8 men leave the job how many trees wiil be cut in 12 hours?
A. 74
B. 82
C. 72
D. 73
ANSWERS AND SOLUTION :
6(C) Explanation
By basic formula:
M1 = 40, D1 = 8, W1 = 60(cutting of trees is taken as work)
M2 = 40 - 8 = 32, D = 12, W2 = ?
Putting the values in the formula
M1 D1 W2 = M2 D2 W1
We have , 40 x 8 x W2 = 32 x 12 x 60
or, W2 = (32 x 12 x 60)/(40 x 8) = 72
7.A can do a piece of work in 4 hours; B and C together can do it in 3 hours, while A and C together can do it in 2 hours. How long will B alone take to do it?
A. 8 hours
B. 10 hours
C. 12 hours
D. 24 hours
ANSWERS AND SOLUTION :
7(C)Explanation:
A's 1 hour's work = 1 /4 ;
(B + C)'s 1 hour's work = 1/3 ;
(A + C)'s 1 hour's work = 1/2 .
(A + B + C)'s 1 hour's work = (1/4 + 1/3) = 7/12
B's 1 hour's work = (7/12 - 1/2) = 1/12 .
So, B alone will take 12 hours to do the work.
8. P and Q can complete a work in 20 days and 12 days respectively. P alone started the work and Q joined him after 4 days till the completion of the work. How long did the work last?
A. 5 days
B. 10 days
C. 14 days
D. 22 days
ANSWERS AND SOLUTION :
8(B)Explanation :
Work done by P in 1 day = 1/20
Work done by Q in 1 day = 1/12
Work done by P in 4 days = 4 × (1/20) = 1/5
Remaining work = 1 – 1/5 = 4/5
Work done by P and Q in 1 day = 1/20 + 1/12 = 8/60 = 2/15
Number of days P and Q take to complete the remaining work = (4/5) / (2/15) = 6
Total days = 4 + 6 = 10
9. If daily wages of a man is double to that of a woman, how many men should work for 25 days to earn Rs.14400? Given that wages for 40 women for 30 days are Rs.21600.
A. 12
B. 14
C. 16D. 18
ANSWERS AND SOLUTION :
9(C)Explanation :
Wages of 1 woman for 1 day = (21600)/(40 x 30)
Wages of 1 man for 1 day = (21600×2)/(40×30)
Wages of 1 man for 25 days = (21600×2×25)/(40×30) = 1080000/1200=900
Number of men = 14400/900 =16
10. Assume that 20 cows and 40 goats can be kept for 10 days for Rs.460. If the cost of keeping 5 goats is the same as the cost of keeping 1 cow, what will be the cost for keeping 50 cows and 30 goats for 12 days?A. Rs.1104B. Rs.1000C. Rs.934D. Rs.1210
ANSWERS AND SOLUTION :
10(A)Explanation :
Assume that cost of keeping a cow for 1 day = c ,
cost of keeping a goat for 1 day = g
Cost of keeping 20 cows and 40 goats for 10 days = 460
Cost of keeping 20 cows and 40 goats for 1 day = 460/10 = 46
=> 20c + 40g = 46
=> 10c + 20g = 23 ---(1)
Given that 5g = c
Hence equation (1) can be written as 10c + 4c = 23 => 14c =23
=> c=23/14
cost of keeping 50 cows and 30 goats for 1 day
= 50c + 30g
= 50c + 6c (substituted 5g = c)
= 56 c = 56×23/14
= 92
Cost of keeping 50 cows and 30 goats for 12 days = 12×92 = 1104
6.If we include the working hours (say T1 and T2) for the two groups then the relationship is
Again, if the efficiency (Say E1 and E2) of the persons in two groups is different then the relationship is
PART - 2
Time : Time needed by more than one person to complete a job or time for which a person(s) actually worked on the assigned job.
Work : The amount of total work or the part of total assigned work actually done.
Note -: In conventional Method work is always treated as 1
Example: So if I say that a person can complete a work in 20 days that means he will do 1/20 work in one day, It's simple maths.
Now another person does the same work in 30 days. So he will do 1/30 work in 1 day.
Now if i ask in how many days they will complete the work together then is Add their 1 day of work
like (1/20 + 1/30) = (3+2)/60 = 5/60 = 1/12
Now this 1/12 we got is actually their 1 day work, So if they do 1/12 work in one day then it's simple they will complete the whole work in 12 days.
Now that was the conventional method and I believe that you all know how to solve Questions through Conventional method.
So now lets move on to the Faster method i.e efficiency method.
In efficiency method the Work is not treated in numerical value, Like in Conventional method the work is 1 but here the work is treated as percentage.
So by common sense the work is always treated as 100%
So when i say a person completes a work in 20 days it means he will do 100/20 % work in 1 day i.e 5 % work in 1 day
If another person does the work in 30 days that means he will do 3.33% work in 1 day.
And together they will do 5 + 3.33 = 8.33 or 8.4% work in one day So in how many days they will do the complete work, that will be 100/8.4 = 12 days.
Now it may sound difficult That we have to convert Each value in % but don't worry you don't have to convert each value, You just have to learn all the values till 1/60 and then it will be a cakewalk.
Now we will take Some standard Cases Of time and work and you all are free to ask any problem if you have in any case.
Case 1 - A does a work in X days, B does a Work in Y days In how many days they will complete the work.
Question- A completes the work in 6 days and B completes the work in 12 days In how many days they will complete the work.
Conventional Method :
Work done by A in 1 day = 1/6
Work Done by B in 1 day = 1/12
Work done By A & B together in 1 day = 1/6 + 1/12 = (2+1)/12 = 3/12 = 1/4
As A & B Completes 1/4 work in one day So they will complete the whole work in 4 Days.
Efficiency method.
Efficiency of A =100/6 = 16.66%
Efficiency of B = 100/12 = 8.33%
Efficiency of A & B Together = 16.66+ 8.33 = 25%
So the time taken by A & B together to Complete the work will be 100/25 = 4 Days.
Case -2 : A can do a work in X days and B can do it Y days, In how many days the work is completed if they work alternatively Started by A.
Now in these type of question the person are not actually working together, what happens in this type of question is that A works for 1 day and then on 2nd day B work and on 3rd again A work and on Fourth again B works and so on till the work is completed.
For example : A can do a work in 10 days B can do it 15 days and how many days they will finish it if The work is started by A
So again work done by A in one day = 1/10
'' '' '' '' '' B '' '' = 1/15
Now the work done by Together will be = 1/10 + 1/15 = 1/6 [ Note now this 1/6 work is not done by them in 1 day but in 2 days Actually, See A worked for 1 day and did 1/10 work on the second day B worked and finished the 1/15 work So in total 2 days they did 1/6 work]
So in 2 days they did 1/6 work so in how many days they will complete the whole work, Simple 12 days.
Efficiency Method :
A's Efficiency = 10%
B's Efficiency = 6.66%
A + B Efficiency = 16.66%
Work done by A and B in 2 days [ remember 2 days because they are not working together but working alternatively] = 16.66%
So time taken by them to complete 100% work = 100/(16.66 = 6 [ but always remember multiply this by 2, Because 16.66% work is done by them in 2 days and not in 1 day.
So The answer will be 6*2 = 12 days.
Case 3: A can do a work in X days, B can do the work Y days and A leaves after working Z days.
Question- A can do a work in 12 days and B can do it in 24 days, A works for 3 days and then leaves, In how many days will the work be completed?
Now here we can so that A leaves after 3 days that means A and B only worked for 3 days and the remaining work is done by B alone.
So first we have to calculated the work done by A and B together in 2 days.
So work done be A in 1 day = 1/12
"" " " " B " " " = 1/24
Work done by A & B together is 1 day = 1/12 + 1/24 = 1/8
Work done by A & B together in 2 days = (1/8) * 3 = 3/8
So remaining work = 1 - 3/8 = 5/8
Now this 5/8 work has to be done by B alone.
So it takes 24 days for B to do Complete work, How much time it will be taken by B to do 5/8 work ? So it will be 24*(5/8) = 15 days
So the work will be completed in 3 + 15 days = 18 days
Efficiency method :
A's efficiency = 8.33 %
B's Efficiency = 4.16%
Total A+B = 12.49% = 12.50%
work done by A and B together in 2 days = 12.5*3 = 37.5 %
Work remaining = 62.5%
time taken by B to complete 62.5% work = 62.50/4.16 = 15 days
Total time = 15+3 = 18 days
Case 4 : A can do a piece of Work in 12 days and B can do it in 24 days, In how many days will the work be completed if B leaves 2 days before the completion on work.
Now in this question B leaves before 2 days
Together they can do the work in what = 1/12 + 1/24 = 1/8
That means 8 Days.
That means Together they could have completed the work in 8 days but B works only till 6th day and The remaining work will be done by A alone
So they worked together for 6 days in all So work done by them in 6 days = (1/8)*6 = 3/4
remaining work = 1/4
Now this 1/4 work will be done by A alone
Now A can do the complete work in 12 days, So the time taken by him to do 1/4 work = 12 * (1/4) = 12/4 days or 3 days
So total time taken = 6+ 3 days = 9 days
Efficiency method :
A's efficiency = 8.33%
B's efficiency = 4.16%
Total = 12.49% = 12.5%
Work will be completed in 8 days
Work done in 6 days = 75%
remaining = 25%
A will complete the remaining in = 25/8.33 = 3
Total = 6+3 = 9 days
Case 5 :.If ‘M1’ persons can do ‘W1’ work in ‘D1’ days and ‘M2’ persons can do W2 work in D2, days then we have a very general formula in the relationship of M1 D1 W2 = M2 D2 W1. The above relationship can be taken as a very basic and all-in-one formula. We also derive
A) More men less days and conversely more days less men.
B) More men more work and conversely more work more men.
C) More days more work and conversely more work more days.
Case 6 : If we include the working hours (say T1 and T2) for the two groups then the relationship is
M1 D1 T1 W2 = M2 D2 T2 W1
Again, if the efficiency (Say E1 and E2) of the persons in two groups is different then the relationship is
M1 D1 T1 E1 W2 = M2 D2 T2 E2 W1
Now, we should go ahead starting with simpler to difficult and more difficult questions.
PART - 3
1. If A and B working together, can finish a piece of work in x days, B and C in y days, C and A in z days, then
(a) A, B and C working together will complete the job in
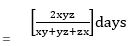

2. (a) If A can complete a work in x days and B is k times efficient than A, then the time taken by both A and B, working together to complete the work is
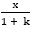 (b) If A and B, working together, can complete a work in x days and B is k times efficient than A, then the time taken by -
(b) If A and B, working together, can complete a work in x days and B is k times efficient than A, then the time taken by -

3. If A working alone takes ‘a’ days more and B working alone takes ‘b’ days more than A and B together, then the number of days taken by A and B, working together, to finish a job is given by 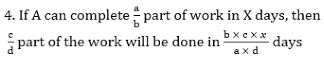


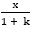

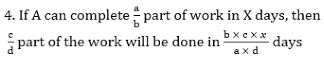

No comments:
Post a Comment